
7.2 Compound Angle Formulas
Helpful Videos
Determine the exact value of sin (π12) using a compound angle formula.
Example 1
Step 1) Resist the urge to pull out your calculator. Instead, let’s try to break π/12 into two special angles. It’s too small for any special angles to add up to it, so we must use a subtraction formula. After playing around withit, you should get the following:
Step 2) Since we have proved that π/12= π/4 - π6, we can now plug those values into a subtraction formula.
π/12= π/4 - π/6
= (3)( π/4) - ( π/6)(2)
= 3 π/12- 2 π/12
= π/12
sin( π/12) = sin( π/4- π/6)
= sin π/4cos π/6 - cos π/4sin π/6
= ( √2/2)( √3/2) - ( √2/2)(1/2)
= √2/2) 34- 24


Simplify sinx cos2x - cosx sin2x
Example 2
Step 1) Recognize this is a compound angle formula (more specifically addition). You can simplify this to the right side of the subtraction formula since we know the values of a and b.
sin (a - b) = sin a cos b - cos a sin b
= sinx cos2x - cosx sin2x.
= sin (x-2x)
= sin -x
Therefore this expression simplifies to sin -x.
Step 2) Simplify the inside of the bracket.
Essentially, we are breaking the angle into two special angles, either by addition or subtraction. This means that the trigonometric ratios of an angle is related to the trigonometric ratios of the angles that add up or subtract to give this angle according to the formulas provided.
Alternatively, we can evaluate the trigonometric ratio of a sum of angles if we have the ratios of each individual angles.
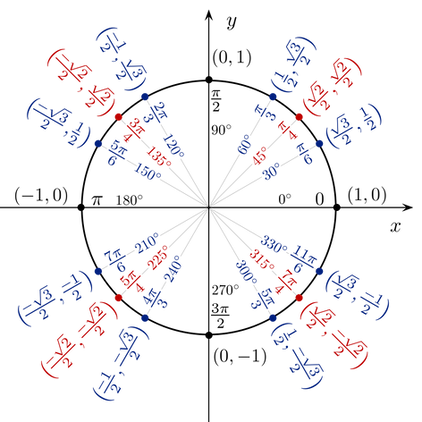
Throughout this chapter, you should be training yourself to complete questions without resorting to your calculators.
What?? No calculators? HOW? That’s probably your reaction right now. But the truth is, you should be relatively happy that you don't really need to use calculators for this chapter because that means you won’t NEED a calculator (so no extra long and ugly calculations).
How can we evaluate trigonometric ratios without using calculators? Simple, we can use special angles (yes, you will have the unit circle at your disposal) which give us the exact value of primary and reciprocal trigonometric ratios.
I know what you’re thinking. How about if we are asked to solve for trigonometric ratios for angles that aren’t special? How can we solve those without calculators if we don’t have exact values for them?
Well, there’s only one way. We have to turn them into angles that we can actually evaluate without calculators.
And this is where compound angle formulas come in. There are two types of compound angle formulas that we will use; addition and subtraction formulas. The following formulas are the formulas that will be used during this chapter.
We can simplify trigonometric expressions by recognizing the compound angle formulas and expressing them as a single angle.
The tutorial below shows step by step how to manipulate these angles and formulas.

Sample Questions
Level 1/2
1. Rewrite each expression as a single trigonometric ratio.
a) sin d cos 2d + cos d sin 2d b) cos 14x cos 11x - sin 14x sin 11x
c) cos a cos b +sin a sin b d) [tan y - tan 3y]/[1 + tanytan3y]
2. Express each expression as a single trigonometric ratio. Evaluate the ratio in exact value.
a) cos 712cos 12 + sin712 sin 12 b) sin 10 cos 35 + cos 10 sin 35
Level 3
3. Determine the exact value of each trigonometric ratio.
a) sin 15° b) cosπ12
4. Using compound angle formulas, create an equivalent expression for each ratio.
a) sin (a - b) b) tan (3x) c) cos (t -2)
Level 4
5.Create a chart which consists of the differences and similarities of compound angle formulas. Include notes which will help you remember how to manipulate them.
Key Concepts/Tips

You can use these equations to obtain exact values for trigonometric ratios as well as use these equations to show that certain expressions are equivalent. This method helps break smaller or more sobscure angles into components and then solve for their exact values without the aid of a calculator
TIP be mindful that some of the exact values will not simplify any further and it imporant to know some multiplication and division rules when trying to simplify the exact value down to its lowest form